Related Resources: heat transfer

Heat Loss From House or Building

Heat Transfer Engineering
HVAC Systems Engineering
Civil Engineering

Heat Loss From House or Building Calculations

Heat loss occurs from a building structure principally due to conduction through exterior surfaces such as walls and windows. Because heat moves in all directions, when calculating the heat loss of a building, we much consider all surfaces (external walls, roof, ceiling, floor, and glass) that divide the inside, heated space from the outside.

The total heat loss from a building (house) may be determined by:

Qtotal = Q(heat loss via walls) + Q(windows) + Q(floor) + Q(ground) + Q(ventilation) +, Q(etc.)....

or

Qtotal = ( ΣΔU · A ) · ΔT

Where

Σ = the sum of..

Qtotal = Total rate of heat loss through walls, roof, glass, etc in Btu/hr
A = Net area of heat loss element (wall, window, etc. ) ft2
U = Overall heat-transfer coefficient of walls, roof, ceiling, floor, or glass in Btu/hr ft2 °F
ΔT = change of temperature in °F

The heat loss through walls is determined by equation:

Q = A · U · ( Ti - To )

Where

Q = Total rate of heat loss through walls, roof, glass, etc in Btu/hr
U = Overall heat-transfer coefficient of walls, roof, ceiling, floor, or glass in Btu/hr ft2 °F
A = Net area of walls, roof, ceiling, floor, or glass in ft2
Ti = Inside design temperature in °F
To = Outside design temperature in °F

Heat Loss from Floors on Slab within a building

Heat loss from floors on slab can be estimated by equation:

Q = F · P · ( Ti - To )

Where: 1)
F = Heat Loss Coefficient for the particular construction in Btu/hr- ft-°F2)
P = perimeter of slab in ft3)
Ti = inside temperature in °F
To = outside temperature in °F

Heat loss from slab-on- grade foundations is a function of the slab perimeter rather than the floor area. Perimeter is the part of the foundation or slab nearest to the surface of the ground outside. The losses are from the edges of the slab and insulation on these edges will significantly reduce the heat losses.

For basement walls, the paths of the heat flow below the grade line are approximately concentric circular patterns centered at the intersection of the grade line and the basement wall. The thermal resistance of the soil and the wall depends on the path length through the soil and the construction of the basement wall. A simplified calculation of the heat loss through the basement walls and floor is given by equation:

Q = A · Ubase · (Tbase – To)

Where

A = Area of basement wall or floor below grade in ft2
Ubase = Overall heat-transfer coefficient of wall or floor and soil path, in Btu/hr ft2 °F
Tbase = basement temperature to be maintained in °F
To = outside temperature in °F

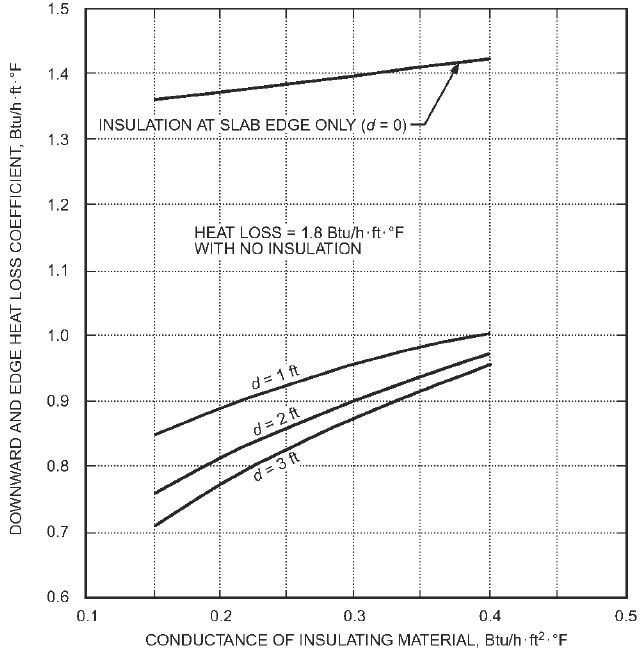
Heat Loss Coefficient for Concrete Floor
Figure 1 - Downward and Edgewise Heat Loss Coefficient for Concrete Floor Slabs on Grade
Courtesy ASHRAE Handbook HVAC Systems and Equipment
Click on image to enlarge

Typical Heat Loss Coefficient of Slab Floor Construction

Construction
Insulation
Btu/h·ft·°F
8 in. block wall, brick facing
Uninsulated
R-5.4 from edge to footer
0.68
0.50
4 in. block wall, brick facing
Uninsulated
R-5.4 from edge to footer
0.50
0.84
Metal stud wall, stucco
Uninsulated
R-5.4 from edge to footer
0.49
1.20
Poured concrete wall with
duct near perimeter*
Uninsulated
R-5.4 from edge to footer
2.12
0.72

*Weighted average temperature of heating duct was assumed at 110ºF during heating season (outdoor air temperature less than 65ºF).

Values of Ubase are roughly given as follows:

Enclosure
0 to 2 ft below grade
Lower than 2 ft
Un insulated wall
0.35
0.15
Insulated wall
0.14
0.09
Basement floor
0.03
0.03

Source: ASHRAE Handbook 1989, Fundamentals

Calculating heat loss through a basement or slab on grade is more difficult for two main reasons: First because the soil can hold a large quantity of heat, second because the temperature in the ground is not the same as outside temperature (in fact it varies little by season). Because of these reasons, buildings loose more heat through their perimeter and the standard practice is to insulate basement walls and 2-4 feet under the slab near those walls. The ASHRAE method is to calculate heat loss for this situation is to look up a perimeter heat loss factor (called "F") in a table based on the "R" value of perimeter insulation used.

Note that the portion of heat transmission from basement is usually neglected unless the weather in winter is severe and the values are significant in comparison with other forms of heat transmission.

Heat Loss Due to Infiltration and Ventilation

The second type of heat loss in buildings is infiltration. To calculate this, you need to know the volume of the space (i.e. sq ft of floor times ceiling height) and how much air typically leaks out , which is often stated as how many times per hour the entire air in the building space is lost to outside and referred to as air changes per hour or ACH. Infiltration can be considered to be 0.15 to 0.5 air changes per hour (ach) at winter design conditions. The more the windows on the external walls, the greater will be the infiltration.

The infiltration/ventilation air quantity estimation is usually done by one of the three methods 1) air change method, 2) infiltration through the cracks and 3) based on occupancy i.e. number of people in the space.

Ventilation rate based on air exchange

V = ACH · A · H / 60

Where

V = Ventilation air in CFM
ACH = Air changes per hour usually 0.15 to 0.5 ACH depending on the construction of the building
A = Area of the space in ft2
H = Height of the room in ft

Note A * H is the volume of the space.

Ventilation rate based on Crack method:

Volume of air = I · A

Where

V = Ventilation air in CFM
I = Infiltration rate usually 0.15 cfm/ft2
A = Area of cracks/openings in ft2

Ventilation rate based on Occupancy method

V = N * 20

Where

V = Ventilation air in CFM o
N = Number of people in space usually 1 person per 100 sq-ft for office application o
20 = Recommended ventilation rate is 20 CFM/person [based on ASHRAE 62 standard for IAQ]

In heat loss estimation, we choose the method that gives the most amount of load.

As soon as the volume flow rate of infiltrated air, CFM, is determined, the sensible heat loss from infiltration can be calculated as

Q = V ·ρair · Cp · (Ti – To) · 60

Where:

Q = sensible is sensible heat load in (Btu/hr)
V = volumetric air flow rate in (cfm)
ρair = density of the air in (Ibm/ft³)
Cp = specific heat capacity of air at constant pressure in (Btu/lbm -F)
Ti = indoor air temperature in (°F)
To = outdoor air temperature in (°F)

Annual Heating Value

The annual heating value is the function of the “degree days” of heating. Heating degree day is defined as a measure of the coldness of the weather experienced. The degree-day concept has traditionally been used to determine the coldness of a climate. When the weather is slightly cool, a little bit of heat might be needed for a few hours in the evening or early morning to stay comfortable. On a very cold day, a lot of heat will be needed all day and all night. A day's average temperature gives some idea of how much heat will be needed on that day. Climatologists use a measurement known as heating degree-days (HDDs) to estimate heating needs more precisely. They assume that people will use at least some heat on any day that has an average outdoor temperature of less than 65ºF. They then calculate the heating needs for each day by subtracting the day's average temperature from 65. The result is the number of heating degrees for that day or HDDs. The higher the number, the more fuel will be used in heating your home or building.

Example for any given day:

High Temp = 50° F
Low Temp = 20° F

Average Temperature = ( 50° + 20° F ) / 2 = 35° F

Degree Day = 65°F - 35° F = 30° F

Therefore, the day was a 30 Degree Day.

From the above data, we can make an educated guess about the annual heat loss. To determine the annual heat loss, divide the energy loss rate by the design temperature difference and then multiply it by 24 hours per day and the number of annual degree days (from the weather files of the location).

For example, a house with a design heating load of 30,000 Btu/hr in Pittsburgh (average temperature of 4°F) will use: [30,000 Btu/hr · 24 hr/day / (65 - 4) (°F)] x 6000 DD/yr = 71million Btu/yr

The concept of degree days is used primarily to evaluate energy demand for heating and cooling services. In the United States, for example, Pittsburgh, Columbus, Ohio, and Denver, Colorado, have comparable annual degree days (about 6000 DD/year). It can be expected that the same structure in all three locations would have about the same heating bill. Move the building to Great Falls, MT (7800 DD/year), it would have a higher heating bill; but in Albuquerque, NM, (4400 DD/year), it would have a relatively lower heating cost.

Although the degree day reading is useful, keep in mind that other factors such as sun load or excessive infiltration due to high wind also affect the heating requirements of a building and are not taken into account by the degree day calculation. We will learn more about the Degree days and the Heat loss estimation in a sample example presented in section-3 of the course but before that let’s briefly discuss the concepts of heat transmission.

Related:

Source

Heat Loss Calculations and Principles, A. Bhatia
ASHRAE HVAC Handbook Systems and Equipment

Link to this Webpage:

Copy Text to clipboard

Spider Optimizer

© Copyright 2000 - 2024, by Engineers Edge, LLC
www.engineersedge.com
All rights reserved
Disclaimer | Feedback
Advertising | Contact